Embark on a captivating journey into the realm of Chapter 9 Stoichiometry Answer Key, where the intricate dance of chemical reactions unfolds. Delve into the fundamental principles that govern the quantitative relationships between reactants and products, unlocking the secrets of balanced equations and precise calculations.

From unraveling the mysteries of mole conversions to exploring the intricacies of gas stoichiometry, this comprehensive guide illuminates the path to mastering the art of chemical proportions. Discover the significance of molarity and dilution in solution stoichiometry, and witness the transformative power of redox reactions.

Stoichiometry Concepts

Stoichiometry is the branch of chemistry that involves the study of the quantitative relationships between the reactants and products in chemical reactions. It helps us predict the amounts of reactants and products involved in a particular reaction.

One of the fundamental principles of stoichiometry is the law of conservation of mass, which states that the total mass of the reactants in a chemical reaction is equal to the total mass of the products. This means that matter cannot be created or destroyed in a chemical reaction, only rearranged.

Another important principle of stoichiometry is the mole concept. A mole is a unit of measurement that represents a specific number of particles (atoms, molecules, ions, or electrons). The mole is defined as the amount of substance that contains exactly 6.022 × 10 ²³particles.

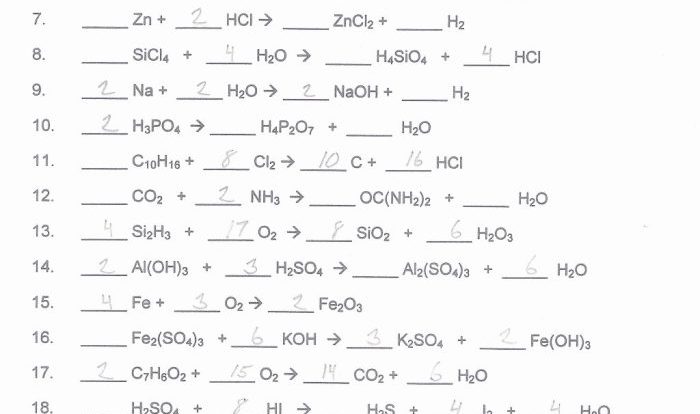
Balancing Chemical Equations

Chemical equations are used to represent chemical reactions. A balanced chemical equation shows the number of atoms of each element on both the reactants and products sides of the equation. Balancing chemical equations is important because it ensures that the law of conservation of mass is obeyed.

To balance a chemical equation, coefficients are added to the reactants and products. Coefficients represent the number of moles of each substance involved in the reaction. The coefficients are adjusted until the number of atoms of each element is the same on both sides of the equation.

Stoichiometric Calculations

Stoichiometric calculations are used to determine the amount of reactants or products that are involved in a chemical reaction. These calculations are based on the balanced chemical equation for the reaction.

To perform a stoichiometric calculation, the following steps are typically followed:

1. Write the balanced chemical equation for the reaction.
2. Convert the given amount of one substance to moles.
3. Use the mole ratio from the balanced chemical equation to convert the moles of the first substance to moles of the second substance.
4. Convert the moles of the second substance to the desired units (mass, volume, etc.).

Mole Calculations

The mole is a fundamental unit in chemistry that represents a specific quantity of a substance. It is defined as the amount of substance that contains exactly 6.02214076 × 10 ²³elementary entities. These entities can be atoms, molecules, ions, or electrons, depending on the substance.

Mole calculations are essential for determining the quantitative relationships between reactants and products in chemical reactions. They allow us to convert between the mass and the number of moles of a substance, which is crucial for stoichiometric calculations.

Converting Between Moles and Grams

The molar mass of a substance is the mass of one mole of that substance. It is expressed in grams per mole (g/mol). To convert between moles and grams, we use the following formula:

Moles = Grams / Molar Mass

Grams = Moles × Molar Mass

Examples of Mole Calculations

Example 1:Calculate the number of moles in 50.0 g of NaCl.

Molar mass of NaCl = 58.44 g/mol

Moles of NaCl = 50.0 g / 58.44 g/mol = 0.856 mol

Example 2:Calculate the mass of 0.250 mol of CaCO ₃.

Molar mass of CaCO ₃= 100.09 g/mol

Mass of CaCO ₃= 0.250 mol × 100.09 g/mol = 25.02 g

Limiting Reactants

In a chemical reaction, the limiting reactant is the reactant that is completely consumed, limiting the amount of product that can be formed. To determine the limiting reactant, we compare the mole ratios of the reactants to the stoichiometric coefficients in the balanced chemical equation.

The limiting reactant is the one with the smallest mole ratio compared to its stoichiometric coefficient.

Gas Stoichiometry

Gas stoichiometry deals with the quantitative relationships between the volumes of gases involved in a chemical reaction. The relationship between the moles of gas and volume is described by the ideal gas law, which states that the volume of a gas is directly proportional to the number of moles of gas, the temperature, and inversely proportional to the pressure.

Relationship between Moles of Gas and Volume

The ideal gas law can be used to calculate the volume of a gas if the number of moles, temperature, and pressure are known. The equation for the ideal gas law is:

“`PV = nRT“`

where:

• P is the pressure of the gas in pascals (Pa)
• V is the volume of the gas in cubic meters (m³)
• n is the number of moles of gas
• R is the ideal gas constant, which is 8.314 J/mol·K
• T is the temperature of the gas in kelvins (K)

This equation can be rearranged to solve for the volume of the gas:

“`V = nRT/P“`

This equation can be used to calculate the volume of a gas if the number of moles, temperature, and pressure are known.

Examples of Gas Stoichiometry Calculations

Gas stoichiometry calculations can be used to solve a variety of problems. For example, they can be used to calculate the volume of a gas that is produced in a chemical reaction, or to calculate the number of moles of gas that are required to react with a given amount of another substance.

One example of a gas stoichiometry calculation is the calculation of the volume of oxygen gas that is produced when 2 moles of propane gas are burned in excess oxygen. The balanced chemical equation for this reaction is:

“`C3H8 + 5O2 → 3CO2 + 4H2O“`

According to this equation, 2 moles of propane gas will react with 5 moles of oxygen gas to produce 3 moles of carbon dioxide gas and 4 moles of water vapor. The volume of oxygen gas that is required for this reaction can be calculated using the ideal gas law:

“`V = nRT/P“`

where:

• n is the number of moles of oxygen gas, which is 5 moles
• R is the ideal gas constant, which is 8.314 J/mol·K
• T is the temperature of the gas, which is assumed to be 298 K
• P is the pressure of the gas, which is assumed to be 1 atm

Plugging these values into the ideal gas law equation, we get:

“`V = (5 moles)

• (8.314 J/mol·K)
• (298 K) / (1 atm) = 112.4 L

“`

Therefore, 2 moles of propane gas will react with 112.4 L of oxygen gas to produce 3 moles of carbon dioxide gas and 4 moles of water vapor.

Concept of Ideal Gases

The ideal gas law is a simplified model of the behavior of gases. It assumes that gas particles are point masses with no volume and that they do not interact with each other. This model is a good approximation for many gases at low pressures and high temperatures.

However, it is not a perfect model, and it can break down at high pressures and low temperatures.

The ideal gas law can be used to make a number of predictions about the behavior of gases. For example, it can be used to predict the volume of a gas at a given temperature and pressure, or to predict the pressure of a gas at a given volume and temperature.

Solution Stoichiometry: Chapter 9 Stoichiometry Answer Key

Solution stoichiometry involves calculations related to the concentrations of solutions. A solution is a homogeneous mixture of two or more substances, where one substance (the solute) is dissolved in another substance (the solvent).

Molarity

Molarity is a measure of the concentration of a solution, defined as the number of moles of solute per liter of solution. It is represented by the symbol M (molar).

Molarity can be calculated using the formula:

“`Molarity (M) = moles of solute / liters of solution“`

Solution Stoichiometry Calculations

Solution stoichiometry calculations involve determining the amount of solute or solvent needed to prepare a solution of a specific concentration.

For example, to prepare 500 mL of a 0.1 M NaCl solution, you would need:

“`Moles of NaCl = Molarity × Volume of solutionMoles of NaCl = 0.1 M × 0.5 LMoles of NaCl = 0.05 moles“`

Then, you would dissolve 0.05 moles of NaCl in 500 mL of water.

Dilution

Dilution is the process of decreasing the concentration of a solution by adding more solvent. The formula for dilution is:

“`M1 × V1 = M2 × V2“`

Where:

• M1 is the initial molarity
• V1 is the initial volume
• M2 is the final molarity
• V2 is the final volume

For example, if you have 100 mL of a 1 M NaCl solution and you want to dilute it to 0.5 M, you would add water until the final volume is 200 mL.

Redox Reactions

Redox reactions are chemical reactions that involve the transfer of electrons between atoms or ions. They are characterized by changes in the oxidation states of the reactants and products.

Oxidation and Reduction, Chapter 9 stoichiometry answer key

Oxidation is the loss of electrons, while reduction is the gain of electrons. An oxidizing agent is a substance that causes another substance to be oxidized, while a reducing agent is a substance that causes another substance to be reduced.

Balancing Redox Equations

Redox equations must be balanced in both mass and charge. The following steps can be used to balance redox equations:

1. Write the unbalanced equation.
2. Identify the oxidizing agent and reducing agent.
3. Balance the equation in terms of mass by adding coefficients to the reactants and products.
4. Balance the equation in terms of charge by adding electrons to the appropriate side of the equation.
5. Check the equation to make sure it is balanced in both mass and charge.

Applications of Stoichiometry

Stoichiometry plays a crucial role in various fields, providing the foundation for understanding and solving problems involving chemical reactions. Its applications extend beyond theoretical chemistry, finding practical significance in engineering, medicine, and other disciplines.

In engineering, stoichiometry is essential for designing chemical processes and optimizing resource utilization. For example, in the chemical industry, stoichiometry helps determine the optimal reactant ratios to maximize product yield and minimize waste. In environmental engineering, stoichiometry aids in understanding and controlling chemical reactions involved in pollution abatement and waste treatment.

Medicine

In medicine, stoichiometry is crucial for developing and administering medications. Understanding the stoichiometry of drug-receptor interactions is vital for determining appropriate dosages and predicting drug efficacy. Stoichiometry also plays a role in understanding the metabolism of drugs within the body, ensuring optimal therapeutic outcomes.

FAQ Summary

What is the significance of balancing chemical equations?

Balancing chemical equations ensures that the number of atoms of each element on the reactants’ side matches the number of atoms of the same element on the products’ side, reflecting the conservation of mass in chemical reactions.

How do I determine the limiting reactant in a reaction?

The limiting reactant is the reactant that is completely consumed in a reaction, limiting the amount of product that can be formed. To identify the limiting reactant, compare the mole ratio of each reactant to its stoichiometric coefficient in the balanced chemical equation.

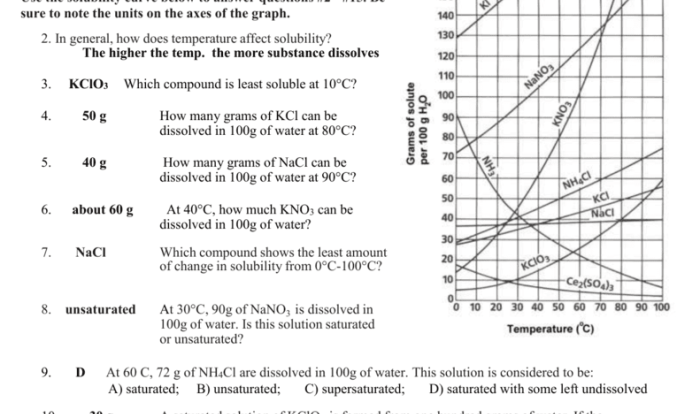
What is the relationship between moles of gas and volume?

Under standard conditions of temperature and pressure (STP), one mole of any gas occupies a volume of 22.4 liters. This relationship is known as the molar volume of a gas.